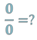
Nothing is something

"Diddly squat is as close to squat as makes no nevermind." (page 124)

"Zero is neither negative nor positive, but the narrowest of no-man's land between those two kingdoms."

(page 190)

This is a superb and far-ranging essay on the apparently mundane zero. While it might be expected to be predominantly mathematical, it is much more, an erudite and masterly exposition that touches many disciplines without slighting its mathematical roots. It has an exponential arc.

Kaplan’s insights and ideas are laid out slowly at first, starting at Almost Nothing and working through the long historical period of zero’s unveiling as the positional placeholder for counting, and zero’s balance point between debits and credits in double entry bookkeeping.

Then, at an ever-increasing rate, mirroring the historical speed of mathematical development, some of zero’s many facets are revealed: As an explicit symbol of nothing , as an infinity (dividing by zero), as the pivot point between positive and negative numbers, as an exponent (always denoting one . . .), as the additive identity, or as indeterminate (0/0), as a recurring element of periodic counting, and as a central part of clever techniques for finding solutions to real polynomials (by arranging an equation to be equal to zero, by including all orders of the polynomial using coefficients of zero for missing terms, by always including the zeroth order term, by arranging an equation as a set of single order terms multiplied by themselves, then solving each term by setting it equal to zero).

The pace increasing again, Kaplan displays the burgeoning power of Almost Nothing, that infinitesimal, those quantities approaching zero, that defines the limit in calculus, which opened up the engineering approach to understanding. He shows the geometrical basis for the development of the derivative, that instantaneous slope of a function, while rapidly deriving the derivative for f(x) = x squared as f'(x) = 2x! Kaplan shows the role of zero in determining the maxima and minima extremes, the presumptive test for which is when a function’s derivative reaches the value of zero, and emphasizes the importance Euler ascribed to the use of these extremes as a key to understanding the world.

Kaplan continues the examination of Almost Nothing with a quick survey of absolute zero, the space-time continuum, special relativity, quantum mechanics, various questions of cosmology, and comes back to zero used as a means for expressing conservation laws and their cousins, equilibrium states (themselves a means for solving systems of difficult dynamic forces by also including the inertial elements as forces to allow an equilibrium to be defined). The pace now frenetic, Kaplan finishes by examining the extensive use of humility, nothingness, worthlessness, and logical negation in philosophy, metaphysics, psychology, religion and linguistics. The author even tosses in a riff on the distinctly American use of zero: We see ourselves as historically unique, starting from nothing.

There is much more here: Any writer who can coherently and cogently blitz through the emptiness of philosophical nothingness, moving from the medieval Sultan Abdul Hamid the 2nd, to McFee’s Casuals of the Sea, to Henry James’ character John Marcher, to Sartre’s Existentialism, to John Bunyan, to Keats, to the Roman Emperor Hadrian, to the poetry of Sylvia Plath, to Dostoyevsky’s character Raskolnikov, to Hermann Weyl, to Ford Madox Ford’s The Good Soldier, all in the course of one five page chapter, is not to be missed!

---

Bonus: A comical take on Nothing and Something in this SNL Bobby Coultsman skit featuring the late great Phil Hartman. The full transcript of this SNL skit here.